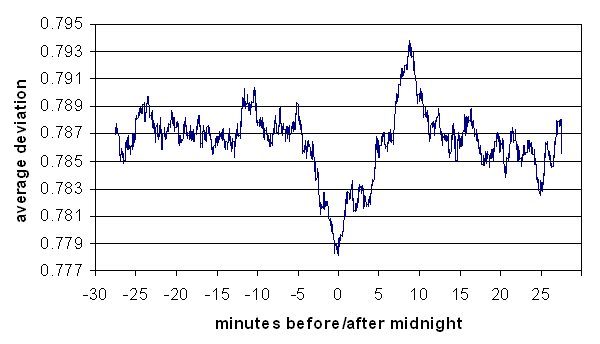
The combined deviation drops precipitously as midnight approaches, and
reaches its extreme minimum at three seconds before midnight, the
moment of transition.
Deviation of normalized variance, GCP Data, Y2K
Other explorations using a related kurtosis
measure (X = e^-kurt)
yielded similar results, buttressed by an extensive permutation analysis
and application of the procedures to data from 1, 2, and 15 days
after the Y2K transition.
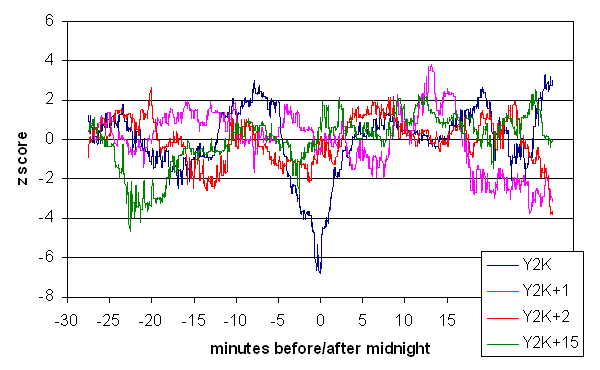
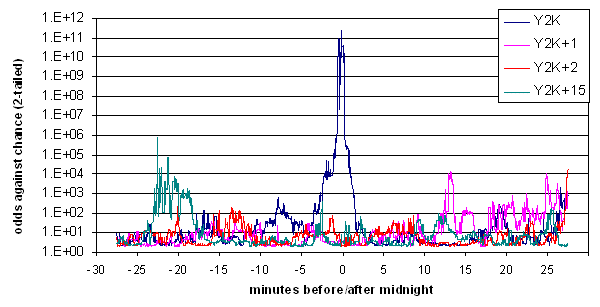
The following two figures display these results, but note, due to a
computation error, the y-axis scale is incorrect and exaggerates the
significance; the actual maximum Z is on the order of 3.5, and the odds
in the second figure have a maximum of about 10e4 or 10e5, not 10e11.
Z-score for Kurtosis Measure, GCP Data at Midnight, Y2K and Controls
Log Odds Against Chance for Kurtosis Measure, Y2K and Controls
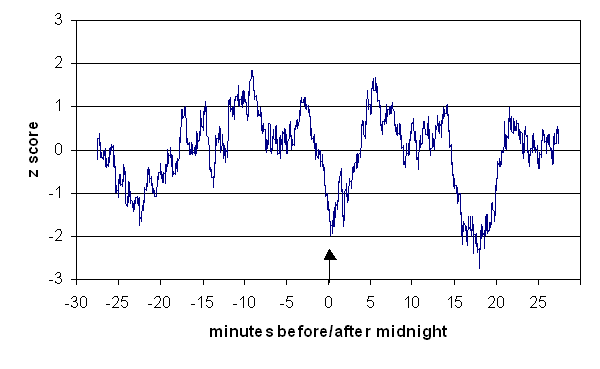
The kurtosis method was applied to the data from the previous
year, for comparison. These data show a somewhat similar structure to
that of the Y2K transition, but the deviation at midnight is somewhat
weaker, and there are competing minima elsewhere.
Z-score, Kurtosis measure, GCP Data, New Years, 1998
Confirmatory Explorations, RDN
The figures representing Dean Radin's analyses
certainly look as if something is going on around
midnight. To gain perspective, I performed several analyses of a
generally similar nature, focusing on the question whether the New
Year's midnight seemed to be correlated with "special" features in the EGG
data. I could not duplicate Dean's analysis exactly (probably
because he used a slightly less complete dataset), but by trying a
variety of analytic modes, found an
impressive array of indicators that parallel his finding and confirm an unusual
feature or tendency just at midnight. The situation is far more complicated
than is obvious, however. Most of these analyses are sensitive to small
changes in the parameters and such factors as the inclusiveness of the
dataset. Although this may suggest that the indicators are artifactual
and selectively chosen, I think there are far too many and that they are
too precisely aligned with the nominal Y2K "target" to be ignored.
Fortunately, the data are available for downloading by any interested
analyst. They bear examination.
Smoothed Variance, 27 eggs, 24 and 36 timezones
This figure shows midnight plus and minus 15 minutes, with data from
27 eggs and 24 timezones. It is a simpler and more direct
implementation of the notion that variability of the data may diminish
near midnight. The figure plots the mean across timezones of the
variance taken across the 27 eggs for
each second, smoothed with a 300-second window. This particular result
looks like a striking confirmation of the hypothesis,
but if the scale is expanded to include ±30 minutes,
another, larger spike appears at 18 minutes after midnight.
Furthermore, if the
number of timezones is increased to 36 so as to include the several half-hour
offset timezones, the picture changes radically. There is no spike at midnight,
but instead, a decreasing trend beginning about 5 minutes before and
ending about 2 minutes after midnight.
Note that this behavior of the variance is entirely consistent with Dean's
prediction, even though it does not maximize at midnight.

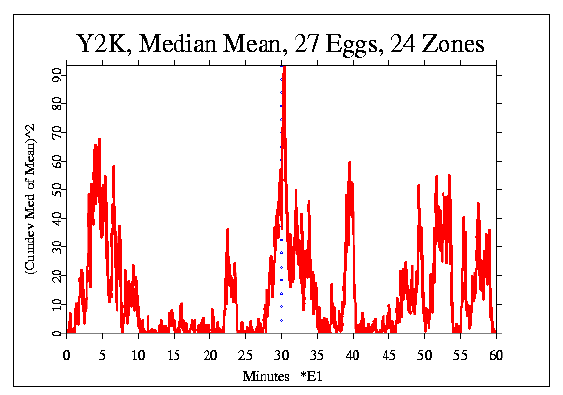
Median Squared Deviation, 27 eggs, 24 and 36 timezones
Another perspective plots changes in the deviation of the mean
trial value across eggs over the time surrounding the Y2K transition.
The cumulative sum of the median of the squared
deviation of the mean from its empirical expectation is plotted,
revealing a striking spike at midnight.
Interpreted literally, this suggests a brief but very sharp increase in
the absolute deviations of trial outcomes just at the moment of greatest
engagement in the New Year's celebration.
Notably, this spike is present with little change in its prominence in
both the 24-timezone and the 36-timezone datasets, shown in the
following two figures. A permutation analysis of the 36-zone case
shows that the maximum
deviation is not extremely rare, with a greater value appearing
somewhere in 90.3% of the permuted datasets. The placement
of the spike so close to midnight (it maximizes at 24 seconds after)
is, however, quite
unlikely; a 4000-permutation analysis yields a p-value of 0.022
of a spike occuring this close or closer to midnight.
The combined probability of a
spike this large and this close is p = 0.020, suggesting that we might
expect to find such impressive structure merely by chance only
twice in 100 repetitions of the Y2K experiment.
The corresponding numbers for the 24-zone dataset are similar, yielding a
combined p-value of 0.017.

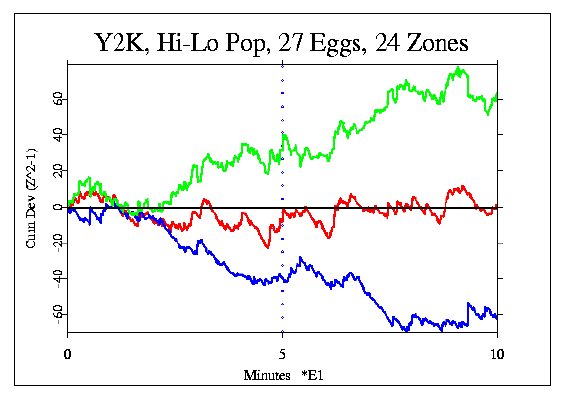
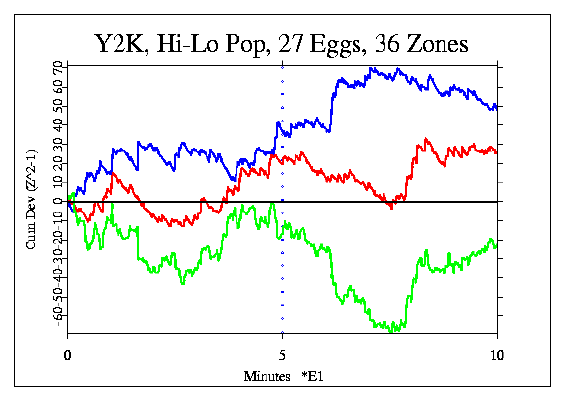
Differences, High and Low Population Zones, 27 eggs
A prediction was made that the high (red curve)and low (blue curve)
population timezones would
show a difference in the cumulative deviations, based on such a finding
last year. The following two figures show this split for 27 eggs across
24 and 36 timezones, respectively.
There is a substantial difference in both cases, but
interpretation is difficult because the direction of the difference is
opposite for 24 and 36 zones,
with the 24 zone dataset showing the predicted positive
difference (green curve), whereas the results for the 36 zone dataset
are opposite to the predicted direction. The difference is significant
in the 24 zone case (Chisquare = 663.29, df = 200, p = 0.037), but is
not significant for the 36 zone dataset (Chisqare = 577.22, df = 600,
p = 0.741).
In parallel with the main prediction, the 36 zone results are used for
the formal results compilation.
Return to top
GCP Home